Questions

May 22, 2009

After receiving a few questions related to the methods described in my first two papers, I thought it might be appropriate to make a kind of question-and-answer page.

What is the trading method exactly?

The method has two components: the main one accumulates shares for the long term while the other accepts short-term trading (long and short). And since you are accumulating shares to hold for the long term, might as well write options on those shares. Idle cash can bear interest.

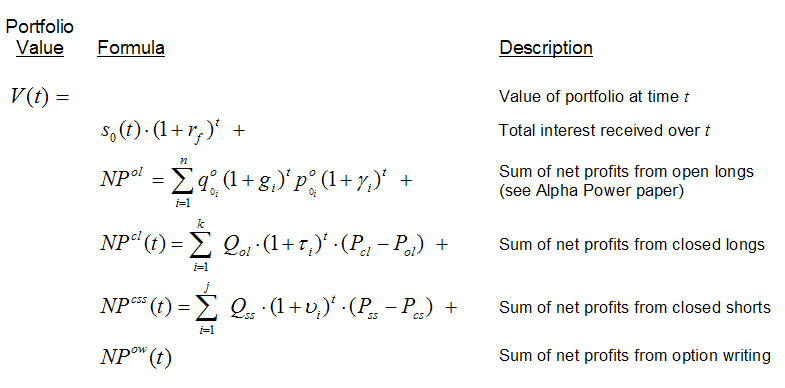
All this is expressed in the formula:

(click to enlarge)

The primary function of the method is to accumulate shares: funds, indexes, ETFs, stocks, whatever you make your pick. Technically, it could be "any" marketable asset that appreciates in time. And since you are trying to accumulate for the long term, might as well select stuff that you think might "live long and prosper", meaning that you expect, long-term, the price to go up.

So, essentially this is an up-market system?

Yes. Over the past 200 years, for the US market, there has not been a single rolling 20-year period that has had negative returns. The bet that maybe in 20 years' time, stocks, on average, will be higher than today has a probability that approaches 1 asymptotically. Like getting close to a sure bet, but with no guarantees. It is not because it never happened in the past that it will not happen in the future. The market has shown examples of this time and time again.

Now say you decide to adopt "this" trading method. For the accumulation side of the equation, you could just Buy & Hold (equivalent to the quantity accumulation rate being zero). If you buy an index, an index fund, or an ETF, and just hold, you become that fund or index. Your expected return is the fund's or index's expected long-term return. We should not be surprised by this, should we?

Isn't this the same methodology used by index funds?

No. An index fund imitates an index by definition. This means that, at all times, the weights of the stocks in the fund will be proportionally close to the weights of the stocks in the index. If the composition of the index does not change, the index fund managers have nothing to do. If the index fund has an inflow (outflow) of cash, it will sit idle or buy (sell) stuff following the market weights. Therefore, they will buy on the way up only when there is sufficient cash inflow and if the market is moving up at the time. Their turnover is very low (little trading; they are of the Buy & Hold trading philosophy), and that is also the main reason why their expenses are low (not much to do).

Then, this method implies that shares will be bought as prices go up?

Yes. Having started this "accumulation program", you also decided to use part of the generated paper profits to progressively buy more of your current holdings as prices are moving up. This does not change the underlying price of the stuff you bought, its progression in time will be the same that you buy more or not. You are kind of doing quasi-random time-volume-price slicing of your trades (I won't go into this at the moment). Nonetheless, having bought more on the way up, you will end up with a greater quantity on hand in the end. And that is the first part of the equation. The price appreciation can be seen as a compounded rate of return, and having the generated profits follow the price, you can opt to accumulate additional holdings at this, or at a fraction of this, growth rate. Your trailing stops will transform some of your intended longer-term trades into shorter-term trades which should keep a major part of their accumulated profits (at least, you should design your trading procedures to do just that).

So what should I expect?

To simplify things, we'll say you buy a single index fund. As time progresses, you accumulate at the index's rate of appreciation. In the long term (20 years), the price should have appreciated somewhere close to a 10% rate, and the quantity on hand at about the same rate. Twenty years at 10 percent per year under the Buy & Hold will be 6.73 times your initial invested capital. And having the quantity increase in time at the same rate will also bring in a factor of 6.73 times your holdings. So to resume, instead of having 6.73 times your initial capital after 20 years in the game, doing nothing but holding, you get 45.26 times your holdings for once in a while buying some more stuff of the stuff you already own as its price is going up. It is not that you will make 6.73 times your capital; it is that you will make 6.73 times the 6.73 times your capital! It is the same result as making 6.73 times the Buy & Hold and is equivalent to a 21% return on your initial capital. Those pennies sure do add up. That's the power of compounding over long periods.

Your premise is to buy high, sell higher?

No, it is not buy high, sell higher. It is buy, buy higher, buy higher, continue to buy higher, and never sell if possible. In essence, you adopt Mr. Buffett's preferred holding period, which is "forever", if reasonable, with the twist of increasing your position with time.

This won't work any better than just buying an index?

No, it is not that this won't work any better than just buying the index. It is, even if you buy an index, simply by reinvesting part of the profits in additional shares, you will outperform the index by a factor equal to your quantity accumulation rate. This is no different from reinvesting dividends. It is only that you systematically apply it to accumulate a larger quantity of the stuff you started with as it goes up in price.

This won't be due to any predictive power but due to the upward drift in stocks?

Yes. Buying an index, you don't even have to make a prediction of where stocks are going, you know that long-term (20 years +) probabilities are on your side that, on average, the price should be somewhat higher. By how much, who knows? I have not seen anyone, or any machine, able to answer that question. But if the trend continues as is (with its 200+ years of history), you should expect an index rate of appreciation somewhere around 10%. It is the highest probable outcome. Can it be something else? Sure, and with high probability, but it will still tend to be 10% from either side. Long-term market trends have a tendency to mean-reverse.

And what does the short-term trading part do in this system?

The short-term trading part is just that: a short-term trading method. It can be any method you wish having a positive expectancy. There is no need to trade if you can't generate, on average, a profit. So this is simply: buy (short) whatever, for whatever reason, and sell (cover) higher (lower). The profits generated are pumped back into the long-term holdings, which will increase further the portfolio's rate of return. Should your trading produce, say, on average, a 10% return per year on your portfolio, and you pump it back in to acquire more shares for the long term, your inventory rate of increase will be about 20%. And this will translate into an overall 32% return on your initial investment or 258 times your initial capital. Again, those pennies do add up.

On the trading side, I recommend starting with small bets that you can increase in time based solely on the profits generated. There is no need to increase the bet size should you not have a real edge. That's what the trading formula says. Once you have established your positive trading edge (long and/or short), you can increase the volume and/or increase trade frequency. Again, that's what the formula says. Either way, you are boosting your profits upward.

You play small bets because the market has a tendency to throw you a curveball here and there. There is always a Lehman or a Madoff somewhere. There is always a WorldCom, an Enron, or a Refco cooking the books in the background, and you never know when one of those will be your preferred high percentage of the portfolio buy-on-the-dip kind of thing. And having a big bet on one of those stocks can destroy your portfolio and put you out of the game. So you place smaller bets as the most basic measure of preservation and portfolio protection. It's the same reason you accept stop-losses as a form of portfolio insurance cost. It is preferable to pay a lot of small insurance fees in order to avoid the big drawdown on the big bet with no other recourse than to accept a portfolio wipeout. I can't put more stress on this than that, we play a treacherous game where on a hundred trades we can make a profit and then on a single trade lose 80% of the portfolio. The risk is too high. I've seen people blow up their entire account on just a single trade in a single day.

This does require that you have found a trading edge?

Yes. The more your trading edge is secure, meaning that it holds in time, the more you can increase the volume (the bet size). And whatever constitutes your edge, should you only participate in a fraction of the time this edge occurs, then you can increase your participation by taking more of such trades. Should you deceive yourself in backtests by doing over-optimization, curve-fitting, or outright peeking, you will find out, at your own expense, that the market does not fool around. From my observation, it has always been ready to massacre any delusion one might have.

So, you say, one can play both long-term and short-term?

Yes. All this is pretty simple, and that is what the trading equations say: make as many small bets as often as your trading edge permits and let the size of your bets grow according to the profits generated. Naturally, at all times, these bets must be marketable and should be kept relatively small compared to the total portfolio.

No matter what you do trading, it will be "all" or "part" of the equation presented above. Should your preference be to trade short-term on the long side only, then only that part of the equation applies to you. The rest has zero value; if you do not hold long-term positions, how can you have long-term profits? Should you always make the same bet, then the rate of increase for the bets is zero. So, your outcome is entirely governed by your average profit per trade, your constant quantity (bet size), and the number of times you can make such a trade. That's fine, the equation still holds.

Playing both long-term and short-term will outperform indexes?

Yes. For those wishing to outperform the indexes and market averages, you have a formula you can follow where your decision process comes into play. On the long-term side, increase the volume and let the market pay for it (my two papers are quite elaborate on this). On the short-term side, find your edge and trade it as often as you possibly can, and as it generates profits, increase the size of your bets and the frequency if you can. Then, take part of the generated profits to buy more long-term holdings, all this within the limits of your available equity at the time. It's a long journey, twenty years and more.

Has anybody been using this type of system?

Well, technically, yes, quite a few and for quite some time. For instance, when you look at Mr. Buffett's long-term record, you can't help but notice that he is following all the components in the equation and more. His preferred holding period is forever. He does use a trailing stop (he does not win all the time). He has made progressively bigger bets in time and he showed he could scale into his positions over months, even years, to outright buying whole companies. He'll take side bets, short-term bets where he knows he has an edge, and pump his accumulated profits into new purchases. Yet, he can withstand 50% drawdowns with a smile knowing that long-term, the market is on his side. His latest bet is a big one: he just bet the farm that in 10 years, the market will be higher than today, and I have to agree with him. He should make very good on this one.

So, to me, the whole equation simply expresses what we can do to optimize performance within the constraints of the account size and the game itself. It is not by adopting the Buy & Hold strategy or only trading your way to a higher portfolio value. It is by doing both and with volume accelerators that you can definitely outperform, in a big way, the market's expected long-term averages.